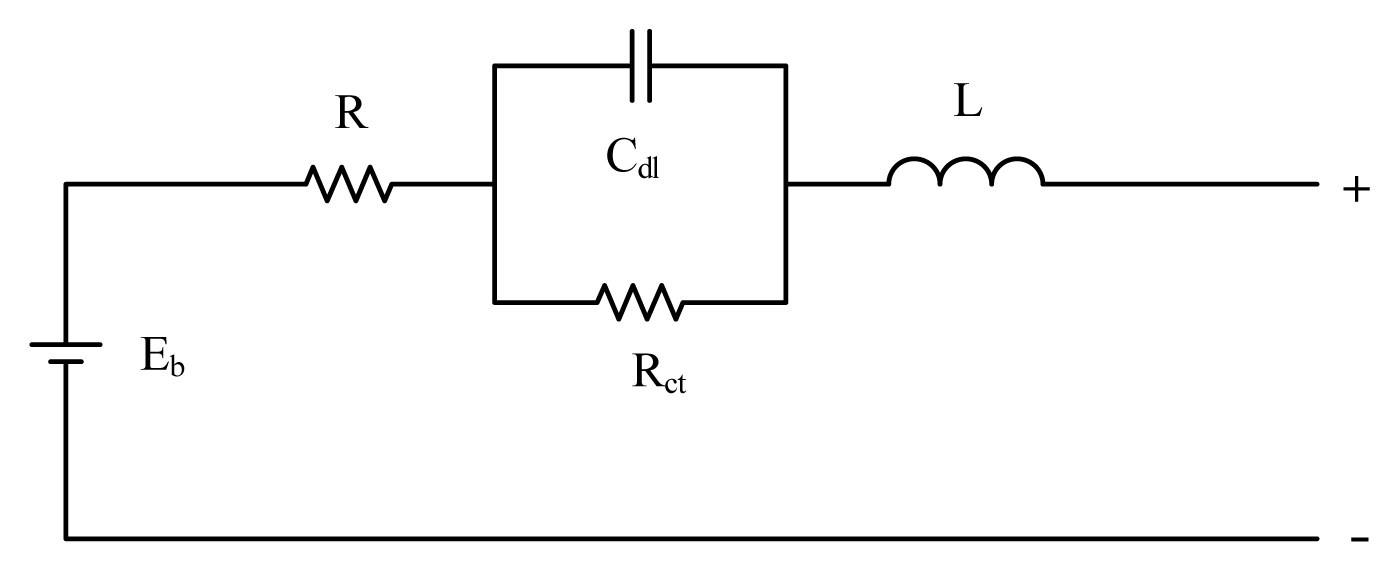
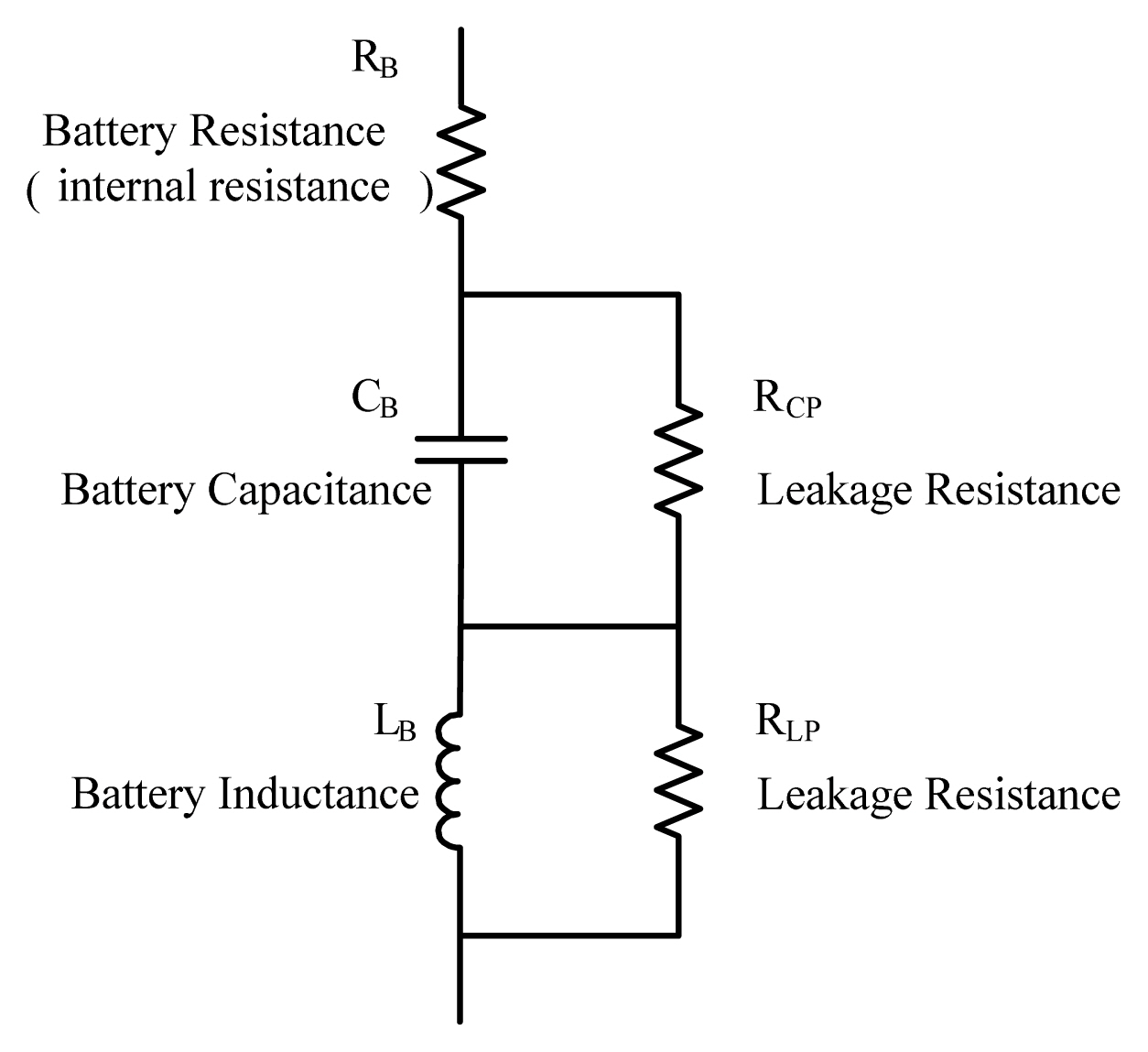
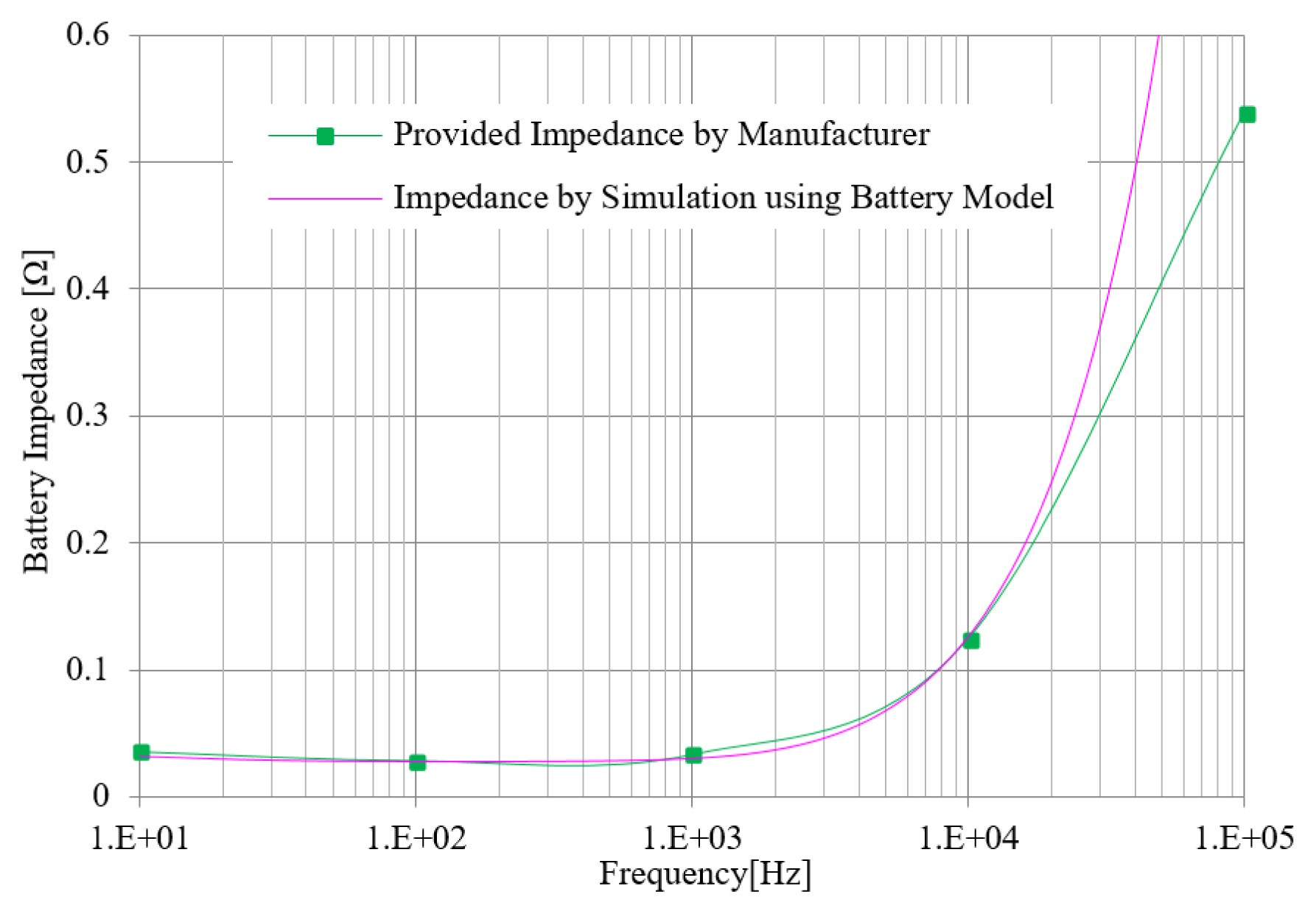
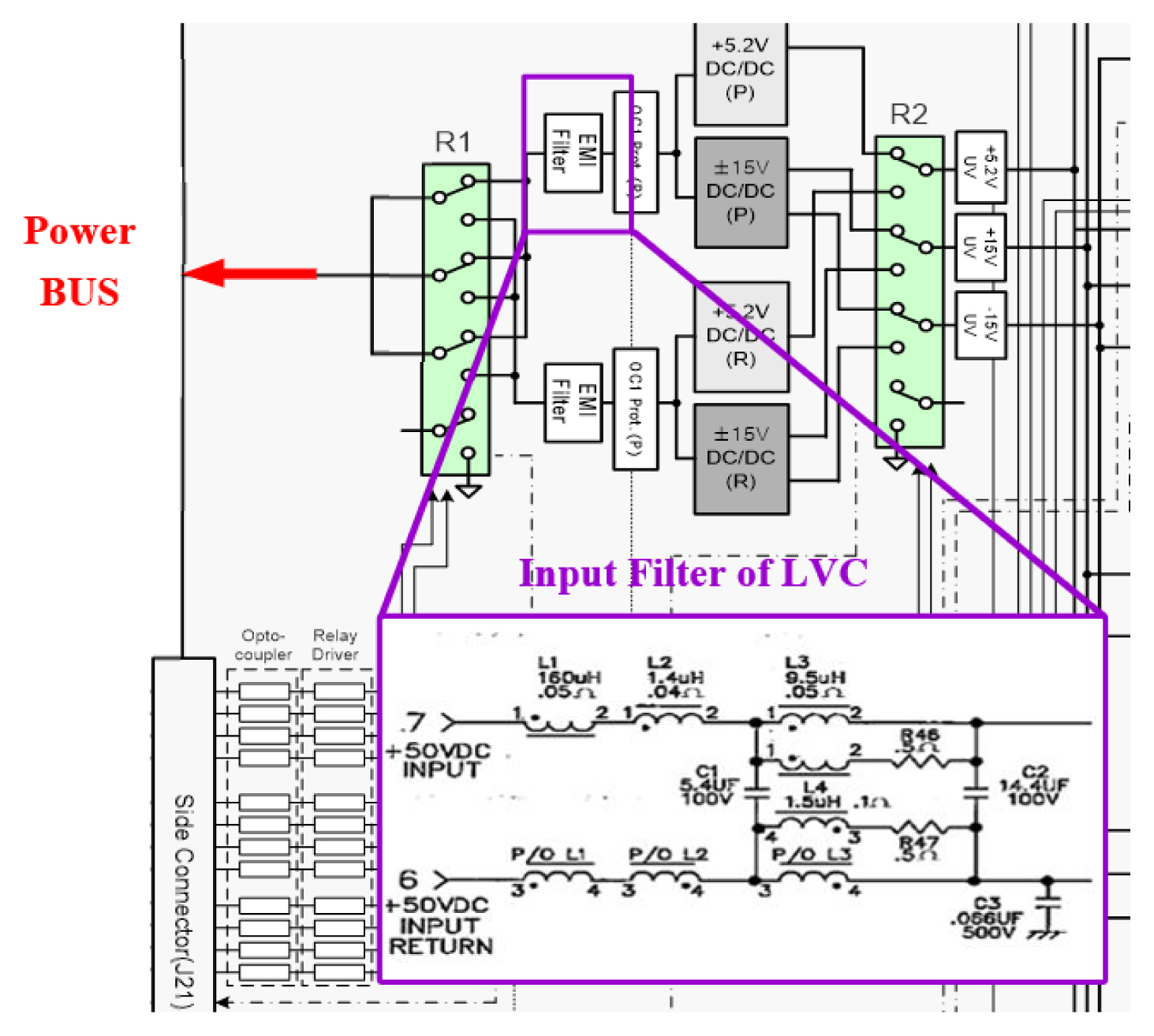
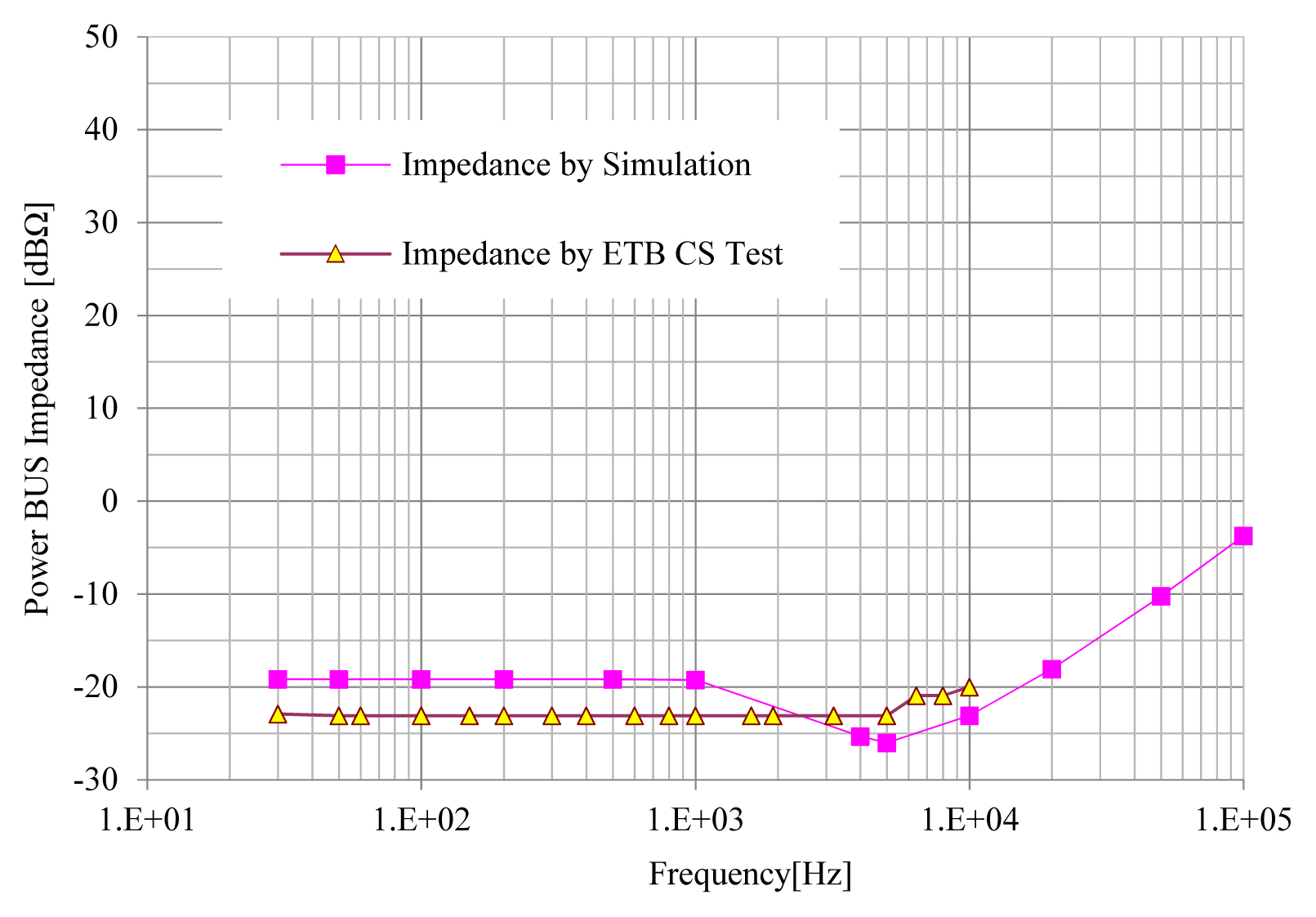
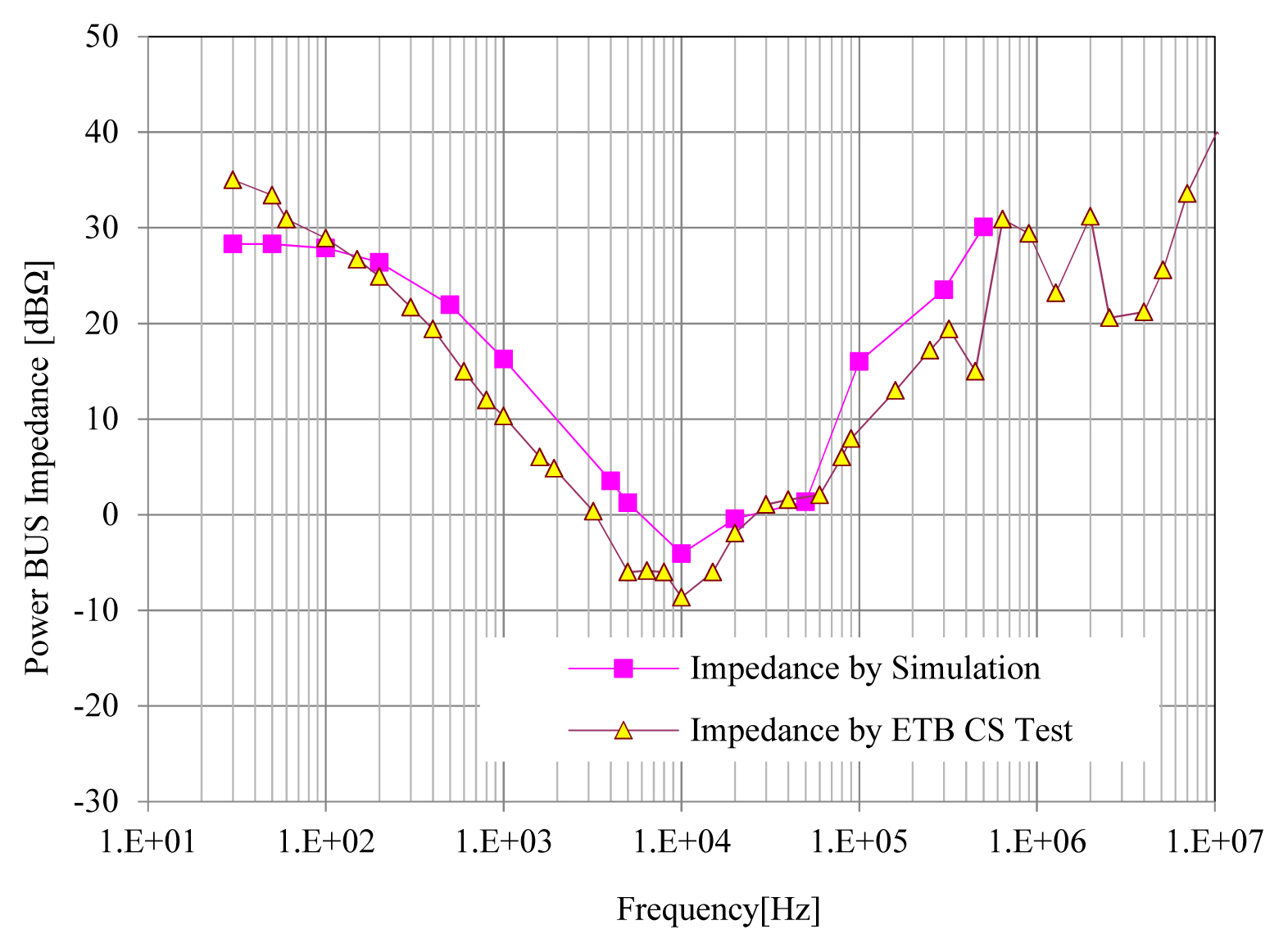
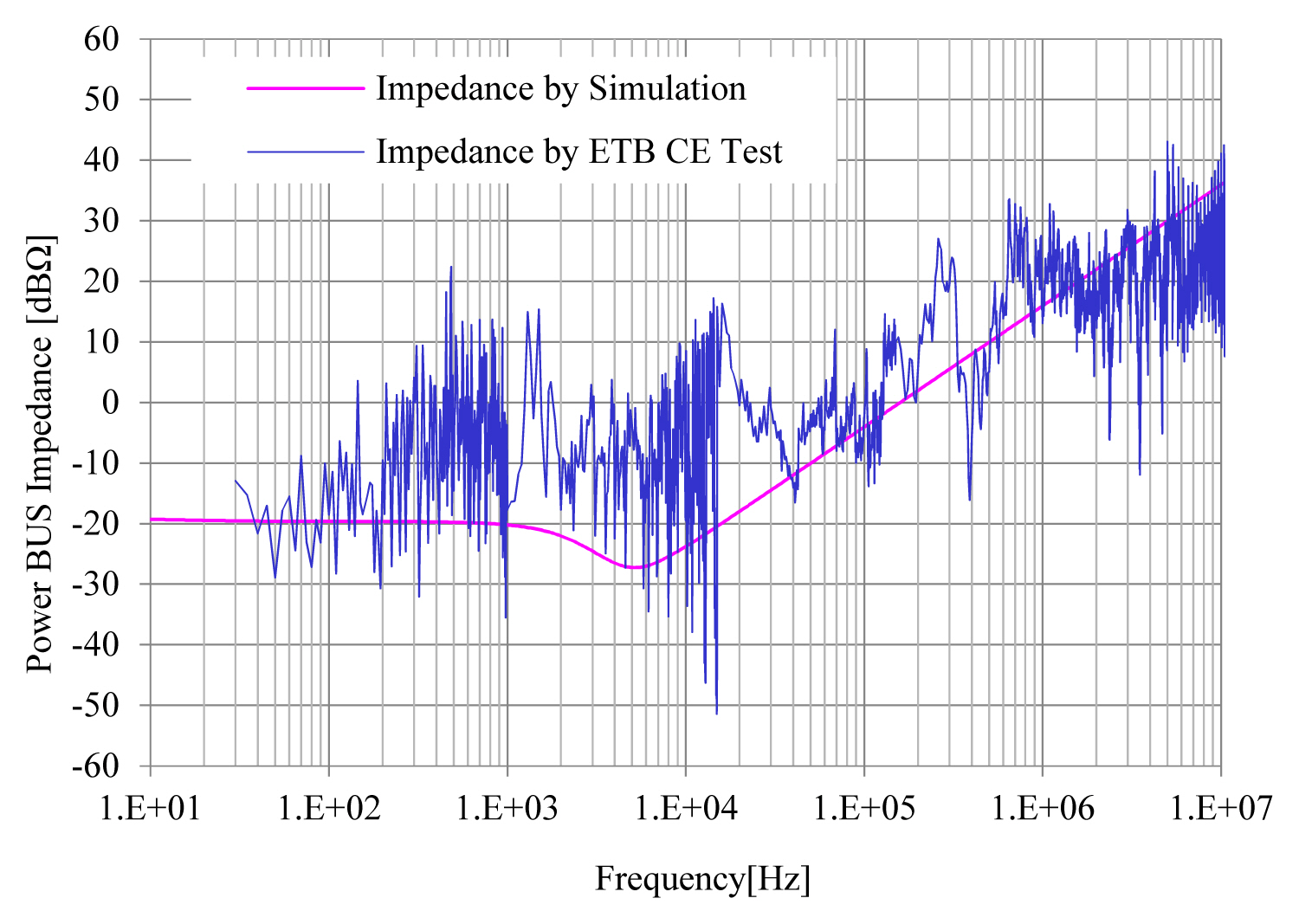
1. I Sharaa, DN Aloi, and HP Gerl, "EMC model-based test-setup of an electrical system,"
Progress In Electromagnetics Research B, vol. 11, pp. 133–154, 2009.

2. G Spadacini, F Grassi, and SA Pignari, "Modelling and simulation of conducted emissions in the powertrain of electric vehicles,"
Progress In Electromagnetics Research B, vol. 69, pp. 1–15, 2016.

3. Z Jiang, RA Dougal, and S Liu, "Application of VTB in design and testing of satellite electrical power systems,"
Journal of Power Sources, vol. 122, no. 1, pp. 95–108, 2003.

4. CS Madhusudhana, GNV Prasad, and CD Sridhara, "Modelling for evaluating efficiency of spacecraft power systems," In: Proceedings of 2014 Power and Energy Systems: Towards Sustainable Energy; Bangalore, India. 2014, pp 64–70.
5. T Mesbahi, N Rizoug, P Bartholomeus, R Sadoun, F Khenfri, and P Le Moigne, "Dynamic model of li-ion batteries incorporating electrothermal and ageing aspects for electric vehicle applications,"
IEEE Transactions on Industrial Electronics, vol. 65, no. 2, pp. 1298–1305, 2018.

6. W Waag, S Kabitz, and DU Sauer, "Experimental investigation of the lithium-ion battery impedance characteristic at various conditions and aging states and its influence on the application,"
Applied Energy, vol. 102, pp. 885–897, 2013.

7. SL Wu, HC Chen, and SR Chou, "Fast estimation of state of charge for lithium-ion batteries,"
Energies, vol. 7, no. 5, pp. 3438–3452, 2014.

8. MS Tabbakh, F Morel, R Mrad, and Y Zaatar, "High frequency battery impedance measurements for EMI prediction," In:
Proceedings of 2013 IEEE International Symposium on Electromagnetic Compatibility; Denver, CO. 2013, pp 763–767.

9. P Pelissou, P Delannoy, X Romeuf, and P Laget, "A high fidelity simulation tool for conducted EMC analysis at system level," In: Proceedings of 2012 ESA Workshop on Aerospace EMC; Venice, Italy. 2012, pp 1–5.
10. DH Baert and AAK Vervaet, "A new method for the measurement of the double layer capacitance for the estimation of battery capacity," In: Proceedings of the 25th International Telecommunications Energy Conference; Yokohama, Japan. 2003, pp 733–738.
11. W Rubel, Satellite design, power system. EADS Astrium GmbH, Bremen, Germany: 2006.
12. CC Huang, JJ Yeh, and CK Tseng, "Estimate filter tank fault effects for satellite power system," In: Proceedings of the World Congress on Engineering; vol. 2, London, UK. 2012, pp 1141–1144.
13. JD Choi, JC Koo, CH Koo, and EC Kim, "Validation of electrical power system using Electrical Test Bed of GEO satellite," In:
Proceedings of 2007 7th International Conference on Power Electronics; Daegu, South Korea. 2007, pp 316–319.