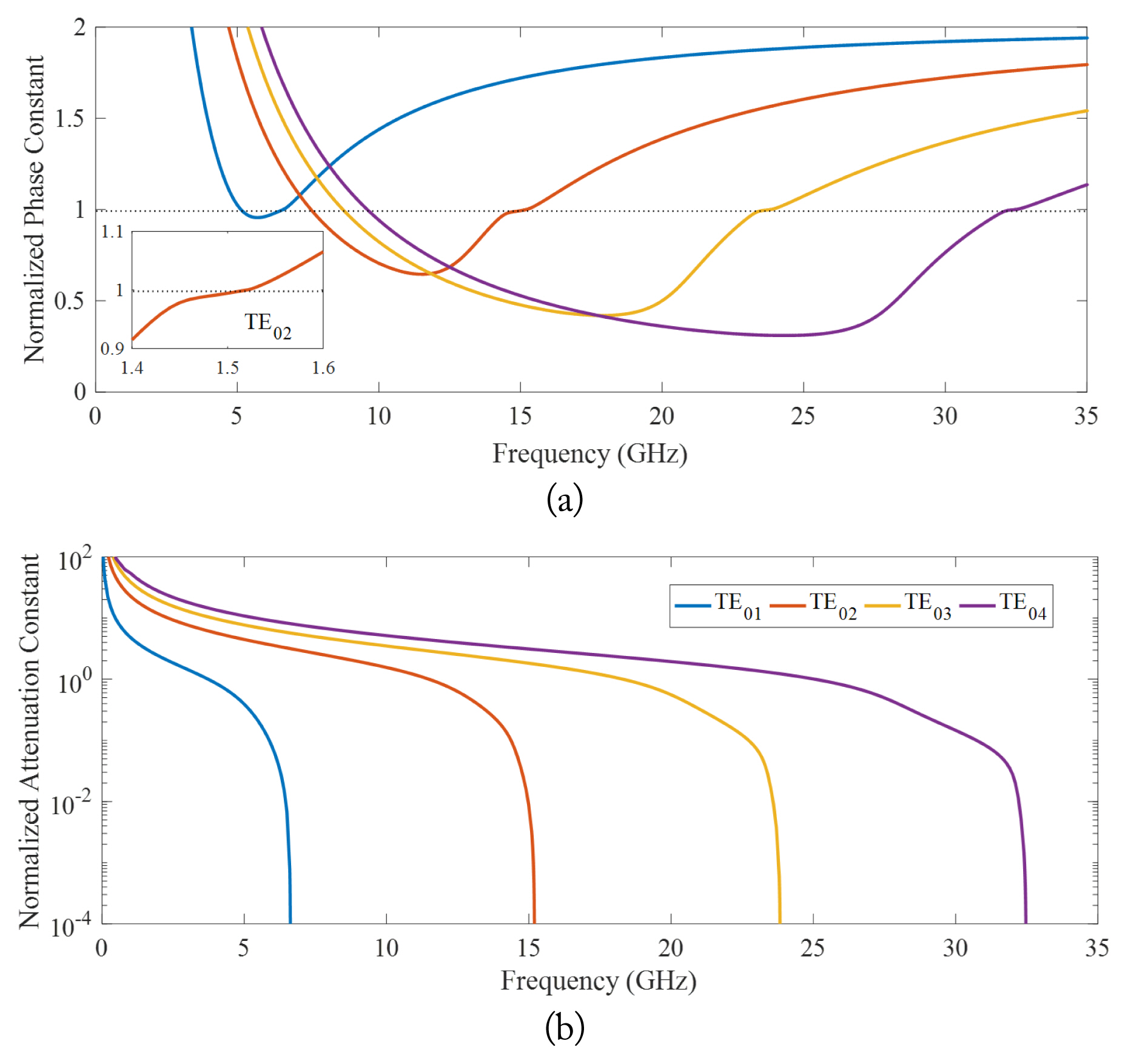
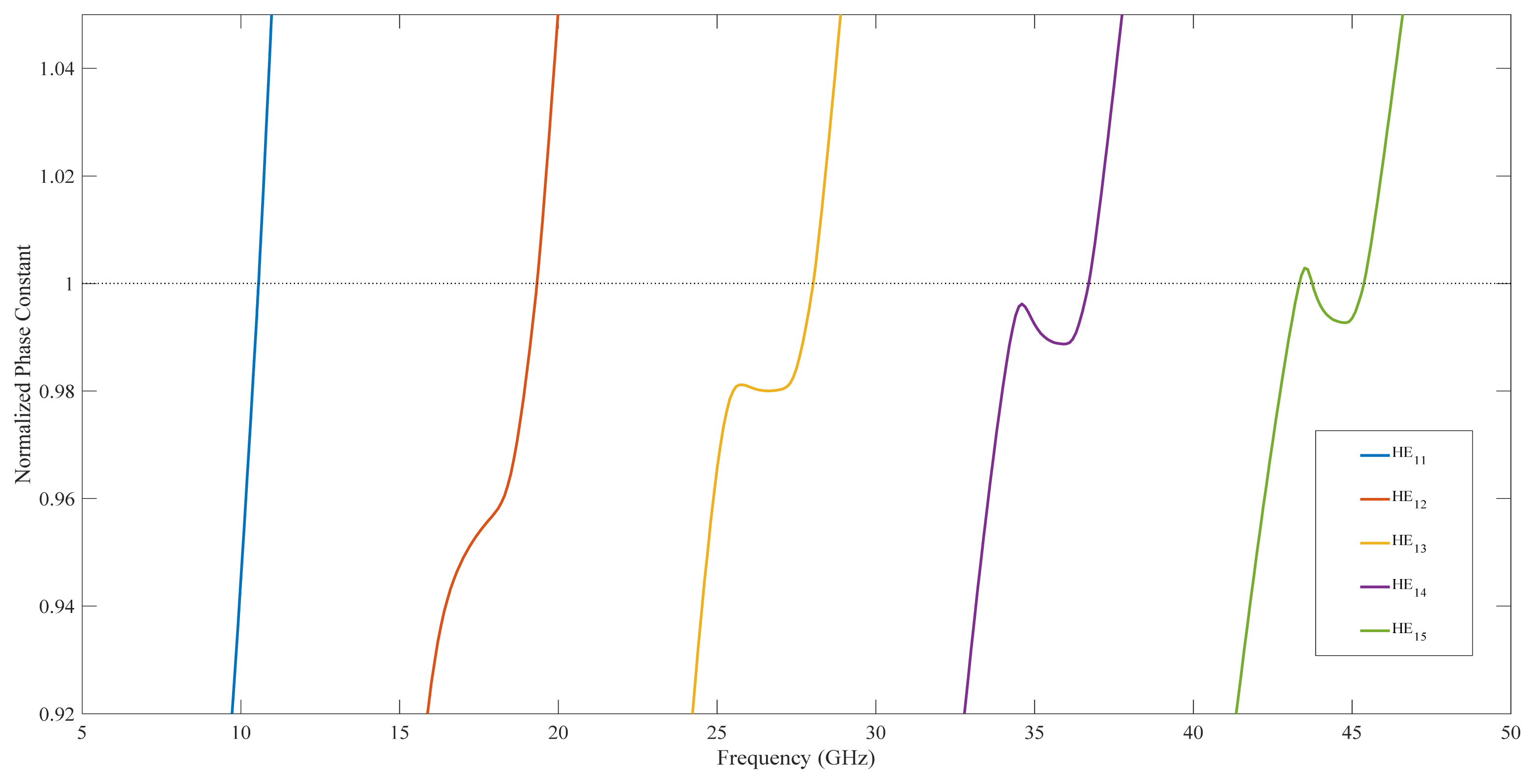
1. SS Yi, "Observation of the scattered TE- and TM-mode coupling in active dielectric cylinder,"
Optics Communications, vol. 173, no. 1–6, pp. 211–215, 2000.

2. M Moisan, A Shivarova, and AW Trivelpiece, "Experimental investigations of the propagation of surface waves along a plasma column,"
Plasma Physics, vol. 24, no. 11, pp. 1331–1400, 1982.

3. H Haus and D Miller, "Attenuation of cutoff modes and leaky modes of dielectric slab structures,"
IEEE Journal of Quantum Electronics, vol. 22, no. 2, pp. 310–318, 1986.

4. Y Yu and L Cao, "Leaky mode engineering: a general design principle for dielectric optical antenna solar absorbers,"
Optics Communications, vol. 314, pp. 79–85, 2014.

5. F Xu and K Wu, "Understanding leaky-wave structures: a special form of guided-wave structure,"
IEEE Microwave Magazine, vol. 14, no. 5, pp. 87–96, 2013.

6. DR Jackson, P Burghignoli, G Lovat, F Capolino, J Chen, DR Wilton, and AA Oliner, "The fundamental physics of directive beaming at microwave and optical frequencies and the role of leaky waves,"
Proceedings of the IEEE, vol. 99, no. 10, pp. 1780–1805, 2011.

7. V Ebrahimi, L Yousefi, and M Mohammad-Taheri, "Enabling inter- and intra-chip optical wireless interconnect by the aid of hybrid plasmonic leaky-wave optical antennas,"
Optics Communications, vol. 382, pp. 119–126, 2017.

8. N Hussain, KE Kedze, and I Park, "Performance of a planar leaky-wave slit antenna for different values of substrate thickness,"
Journal of Electromagnetic Engineering and Science, vol. 17, no. 4, pp. 202–207, 2017.

9. P Burghignoli, W Fuscaldo, D Comite, P Baccarelli, and A Galli, "Higher-order cylindrical leaky waves—Part I: Canonical sources and radiation formulas,"
IEEE Transactions on Antennas and Propagation, vol. 67, no. 11, pp. 6735–6747, 2019.

10. F Monticone and A Alu, "Leaky-wave theory, techniques, and applications from microwaves to visible frequencies,"
Proceedings of the IEEE, vol. 103, no. 5, pp. 793–821, 2015.

11. J Wan, O Rybin, and S Shulga, "Far field focusing for a microwave patch antenna with composite substrate,"
Results in Physics, vol. 8, pp. 971–976, 2018.

12. G Lu, FM Sabirova, and AV Morozov, "Simulation and experimental measurement of digital multi-beamforming phased antenna array in the frequency range C,"
Results in Physics, vol. 13, article no 102310, 2019.
https://doi.org/10.1016/j.rinp.2019.102310

13. AM Tamim, MRI Faruque, MJ Alam, SS Islam, and MT Islam, "Split ring resonator loaded horizontally inverse double L-shaped metamaterial for C-, X-and Kuband microwave applications,"
Results in Physics, vol. 12, pp. 2112–2122, 2019.

14. N Berahim, IS Amiri, T Anwar, SR Azzuhri, MM Nasir, R Zakaria et al., "Polarizing effect of MoSe2-coated optical waveguides,"
Results in Physics, vol. 12, pp. 7–11, 2019.

15. NK Mishra, S Das, and DK Vishwakarma, "Beam steered linear array of cylindrical dielectric resonator antenna,"
AEU-International Journal of Electronics and Communications, vol. 98, pp. 106–113, 2019.

16. A Bostani and H Oraizi, "Analysis and design of radially inhomogeneous tapered dielectric rod antennas,"
AEU-International Journal of Electronics and Communications, vol. 101, pp. 106–113, 2019.

17. ST Yang and H Ling, "Application of compressive sensing to two-dimensional radar imaging using a frequency-scanned microstrip leaky wave antenna,"
Journal of Electromagnetic Engineering and Science, vol. 17, no. 3, pp. 113–119, 2017.

18. P Ranjan and RK Gangwar, "Probe feed half split cylindrical dielectric resonator antenna for wideband application,"
AEU-International Journal of Electronics and Communications, vol. 69, no. 11, pp. 1709–1714, 2015.

19. J Zhu and J Lou, "High-sensitivity Fano resonance temperature sensor in MIM waveguides coupled with a polydimethylsiloxane-sealed semi-square ring resonator,"
Results in Physics, vol. 18, article no 103183, 2020.
https://doi.org/10.1016/j.rinp.2020.103183

20. G Das, NK Sahu, and RK Gangwar, "Dielectric resonator based multiport antenna system with multi-diversity and built-in decoupling mechanism,"
AEU-International Journal of Electronics and Communications, vol. 119, article no 153193, 2020.
https://doi.org/10.1016/j.aeue.2020.153193

21. T Ramachandran, MRI Faruque, and MT Islam, "A dual band left-handed metamaterial-enabled design for satellite applications,"
Results in Physics, vol. 16, article no 102942, 2020.
https://doi.org/10.1016/j.rinp.2020.102942

22. W Su, Y Liu, and B Chen, "Multiple Fano resonances in asymmetric rectangular ring resonator based on graphene nanoribbon,"
Results in Physics, vol. 17, article no 103121, 2020.
https://doi.org/10.1016/j.rinp.2020.103121

23. IS Amiri, T Anwar, R Zakaria, and P Yupapin, "TE-like mode analysis of microsystem InGaAsP/InP semiconductor resonator generating 20 GHz repetition rate pulse trains,"
Results in Physics, vol. 10, pp. 980–986, 2018.

24. IS Amiri, F Alizadeh, MM Ariannejad, R Amini, and P Yupapin, "Computation of ion exchange buried microring resonator waveguide for THz communication applications,"
Results in Physics, vol. 10, pp. 287–290, 2018.

25. MM Ariannejad, IS Amiri, SRB Azzuhri, R Zakaria, and P Yupapin, "Polarization dependence of SU-8 micro ring resonator,"
Results in Physics, vol. 11, pp. 515–522, 2018.

26. X Lu, GG Zheng, and P Zhou, "High performance refractive index sensor with stacked two-layer resonant waveguide gratings,"
Results in Physics, vol. 12, pp. 759–765, 2019.

27. B Zeng, H Xu, M Zhao, C Xiong, C Liu, and H Li, "Optical tunable modulator based on graphene metamaterials at terahertz communication range,"
Results in Physics, vol. 16, article no 102935, 2020.
https://doi.org/10.1016/j.rinp.2020.102935

28. P Wu, Z Chen, H Jile, C Zhang, D Xu, and L Lv, "An infrared perfect absorber based on metal-dielectric-metal multi-layer films with nanocircle holes arrays,"
Results in Physics, vol. 16, article no 102952, 2020.
https://doi.org/10.1016/j.rinp.2020.102952

29. J Hu and CR Menyuk, "Understanding leaky modes: slab waveguide revisited,"
Advances in Optics and Photonics, vol. 1, no. 1, pp. 58–106, 2009.

30. AA Oliner, "Leakage from higher modes on microstrip line with application to antennas,"
Radio Science, vol. 22, no. 6, pp. 907–912, 1987.

31. P Lampariello, F Frezza, and AA Oliner, "The transition region between bound-wave and leaky-wave ranges for a partially dielectric-loaded open guiding structure,"
IEEE Transactions on Microwave Theory and Techniques, vol. 38, no. 12, pp. 1831–1836, 1990.

32. KY Kim, "Guided and leaky modes of circular open electromagnetic waveguides: dielectric, plasma, and metamaterial columns," Ph.D. dissertation, Kyungpook National University. Daegu, Korea, 2004.
33. XY Zeng, SJ Xu, K Wu, and KM Luk, "Properties of guided modes on open structures near the cutoff region using a new version of complex effective dielectric constant,"
IEEE Transactions on Microwave Theory and Techniques, vol. 50, no. 5, pp. 1417–1424, 2002.

34. YD Lin, JW Sheen, and CC Tzuang, "Analysis and design of feeding structures for microstrip leaky wave antenna,"
IEEE Transactions on Microwave Theory and Techniques, vol. 44, no. 9, pp. 1540–1547, 1996.

35. YD Lin and JW Sheen, "Mode distinction and radiation- efficiency analysis of planar leaky-wave line source,"
IEEE Transactions on Microwave Theory and Techniques, vol. 45, no. 10, pp. 1672–1680, 1997.

36. KY Kim, HS Tae, and JH Lee, "Leaky dispersion characteristics in circular dielectric rod using Davidenko’s method," Journal of the Korea Electromagnetic Engineering Society, vol. 5, no. 2, pp. 72–79, 2005.
37. RR Hirani, SK Pathak, SN Shah, and DK Sharma, "Dispersion characteristics of dielectric tube waveguide loaded with plasma for leaky wave antenna application,"
AEU-International Journal of Electronics and Communications, vol. 83, pp. 123–130, 2018.

38. KA Michalski and D Zheng, "Rigorous analysis of open microstrip lines of arbitrary cross-section in bound and leaky regimes,"
IEEE Transactions on Microwave Theory and Techniques, vol. 37, no. 12, pp. 2005–2010, 1989.

39. JS Bagby, CH Lee, DP Nyquist, and Y Yuan, "Identification of propagation regimes on integrated microstrip transmission lines,"
IEEE Transactions on Microwave Theory and Techniques, vol. 41, no. 11, pp. 1887–1894, 1993.

40. C Luxey and JM Laheurte, "Simple design of dual-beam leaky-wave antennas in microstrips,"
IEE Proceedings- Microwaves, Antennas and Propagation, vol. 144, no. 6, pp. 397–402, 1997.

41. KY Kim, HS Tae, and JH Lee, "Analysis of leaky modes in circular dielectric rod waveguides,"
Electronics Letters, vol. 39, no. 1, pp. 61–62, 2003.

42. CA Balanis, Advanced Engineering Electromagnetics. Hoboken, NJ: John Wiley & Sons, 1989.
43. C Yeh and FI Shimabukuro, The Essence of Dielectric Waveguides. Boston, MA: Springer, 2008.
44. C Yeh, "Guided-wave modes in cylindrical optical fibers,"
IEEE Transactions on Education, vol. 30, no. 1, pp. 43–51, 1987.

45. E Snitzer, "Cylindrical dielectric waveguide modes,"
Journal of the Optical Society of America, vol. 51, no. 5, pp. 491–498, 1961.

46. K Morishita, "Hybrid modes in circular cylindrical optical fibers,"
IEEE Transactions on Microwave Theory and Techniques, vol. 31, no. 4, pp. 344–350, 1983.

47. WM Bruno and WB Bridges, "Flexible dielectric waveguides with powder cores,"
IEEE Transactions on Microwave Theory and Techniques, vol. 36, no. 5, pp. 882–890, 1988.

48. H Talisa, "Application of Davidenko’s method to the solution of dispersion relations in lossy waveguiding systems,"
IEEE Transactions on Microwave Theory and Techniques, vol. 33, no. 10, pp. 967–971, 1985.

49. HAN Hejase, "On the use of Davidenko’s method in complex root search,"
IEEE Transactions on Microwave Theory and Techniques, vol. 41, no. 1, pp. 141–143, 1993.

50. K Naishadham and LB Felsen, "Dispersion of waves guided along a cylindrical substrate-superstrate layered medium,"
IEEE Transactions on Antennas and Propagation, vol. 41, no. 3, pp. 304–313, 1993.

51. K Naishadham and HW Yao, "An efficient computation of transient scattering by a perfectly conducting cylinder,"
IEEE Transactions on Antennas and Propagation, vol. 41, no. 11, pp. 1509–1515, 1993.

52. MM Shabat, "Numerical algorithms for modeling hybrid surface plasmon polaritons guided by metal films,"
Microwave and Optical Technology Letters, vol. 16, no. 2, pp. 122–124, 1997.

53. MM Shabat, D Jager, NM Barakat, G Strip, and P Authority, "Numerical and analytical solutions of dispersion equation in lossy nonlinear waveguiding system,"
Microwave and Optical Technology Letters, vol. 22, no. 4, pp. 273–278, 1999.

54. MM Shabat, "Application of Davidenko’s method to a lossy nonlinear waveguide," International Journal of Infrared and Millimeter Waves, vol. 20, no. 7, pp. 1363–1370, 1999.
55. MM Shabat, YS Madi, and D Jager, "Solution of lossy dielectric moving waveguides using numerical method (Davidenko’s approach),"
Electromagnetics, vol. 22, no. 8, pp. 659–666, 2002.

56. Y Liu and T Itoh, "Control of leakage in multilayered conductor-backed coplanar structures," In:
Proceedings of 1994 IEEE MTT-S International Microwave Symposium Digest (Cat No 94CH3389-4); San Diego, CA. 1994, pp 141–144.

57. D Nghiem, JT Williams, DR Jackson, and AA Oliner, "Suppression of leakage on stripline and microstrip structures," In:
Proceedings of 1994 IEEE MTT-S International Microwave Symposium Digest (Cat No 94CH3389- 4); San Diego, CA. 1994, pp 145–148.