|
 |
- Search
| J. Electromagn. Eng. Sci > Volume 21(5); 2021 > Article |
|
Abstract
A new design method for RLC reactive absorbers is presented. This method is based on closed-form solutions to help realize the widest absorption bandwidth for an arbitrarily specified thickness. The solutions for the RLC values of the reactive screen are derived using an equivalent circuit in which the thickness of the substrate used, the permittivity and tangential loss of the substrate, and the frequency are all considered. A perfect match and maximum bandwidth at a design frequency can be achieved with the proposed method. Various aspects of the absorber characteristics, depending on the thickness and loss of the substrate, are analyzed using the presented solutions and electromagnetic (EM) simulations. To validate the proposed design method, an X-band microwave absorber with a crossed-dipole structure patterned on a silver nanowire resistive film is designed, fabricated, and measured. The substrate electrical thickness of the absorber is 70° at 10 GHz, with a permittivity of 2.2. The 90% absorption bandwidth is 8 GHz in the frequency range of 8.2–16.2 GHz. The measured absorption agrees well with the results obtained using circuit and EM simulations.
Microwave absorbers have been widely used for practical applications, such as stealth combat planes, filters, and electromagnetic (EM) interference shields. Numerous studies have been performed on thin and wideband absorbers. The simplest absorber is the Salisbury absorber, which has a resistive sheet of 377 Ω/square placed on a quarter wavelength λ/4 from a ground plane [1–3]. Reactive RLC-type absorbers with the same quarter-wave thickness have been studied using various theoretical approaches and experimental validations [4–6].
Simple closed-form solutions for microwave absorbers with a certain absorption rate at any frequency are presented in [5]. However, the closed-form solutions in [5] can only be applied to absorbers with a quarter wavelength thickness. The condition in which their thickness must be a quarter wavelength λ/4 often results in a bulky absorber, which is not satisfactory for most applications, especially when the required design frequencies are low.
The design of thin but broadband microwave absorbers is an open problem, and many studies have been conducted on it. Some methods have been examined to achieve these goals by adopting multilayer [7, 8], multi-resonance [9–11], magnetic materials [12–14], frequency selective surfaces (FSS) [11, 15–18], and metamaterials (MM) [19–22]. Although multilayered and/or multi-resonance techniques are useful methods to enhance the bandwidth, they have the disadvantages of thickness and size as well as difficulty in fabrication due to the complexity of the structures [7]. A wide range of magnetic materials are used for thin radar absorbers. However, maintaining the uniformity of the material in the microwave band is usually difficult [13]. Recently, single-layer FSS-MM absorbers have been studied. One FSS-MM absorber has been proposed, with a thickness of 2 mm and an absorptivity of more than 90% from 8.5 to 16.0 GHz [20]. An ultrathin and ultra-wideband MM absorber based on periodically arranged metallic square spirals is presented in [21]. It has an absorptivity of more than 90%, from 11.4 to 20 GHz, and a thickness of 1.6 mm (λ/16). The results of this work have not been reproduced in our EM simulations, and a more rigorous validation based on the power conservation law is required.
In this paper, closed-form formulas for the design of a single-layer wideband microwave absorber are proposed. The formulas can be applied to any frequency, electrical thickness, tangential loss, and permittivity of a substrate material. Using the proposed solutions, the RLC value of a reactive screen with an arbitrary thickness can be extracted. These values are used to realize the structure on the screen. A simple crossed-dipole structure, which takes into account the relatively easy optimization of the structure and the lesser dependence of absorption characteristics on the polarization of incident waves, is used for the reactive RLC screen. In Section II, the problem of realizing a thin (less than a quarter wavelength) and wideband absorber is formulated to obtain the exact RLC values based on an equivalent circuit. A convenient guideline for determining the dimensions of the dipole structure is presented. In Section III, a wideband microwave absorber at 10 GHz is designed and fabricated using a silver nanowire (AgNW) resistive film. The theoretical bandwidth of the presented absorber is compared with the measured bandwidth. The study is concluded in Section IV.
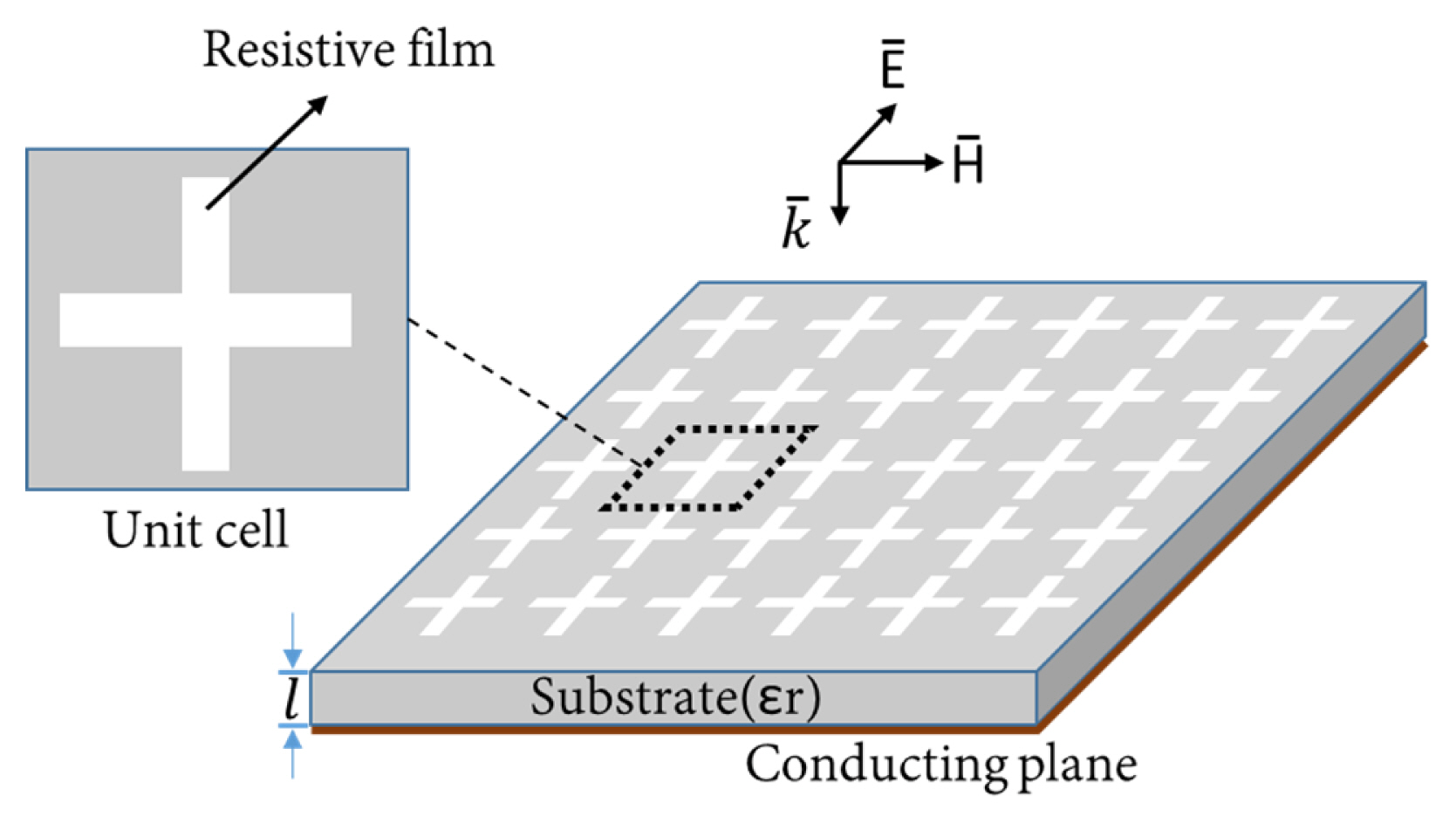
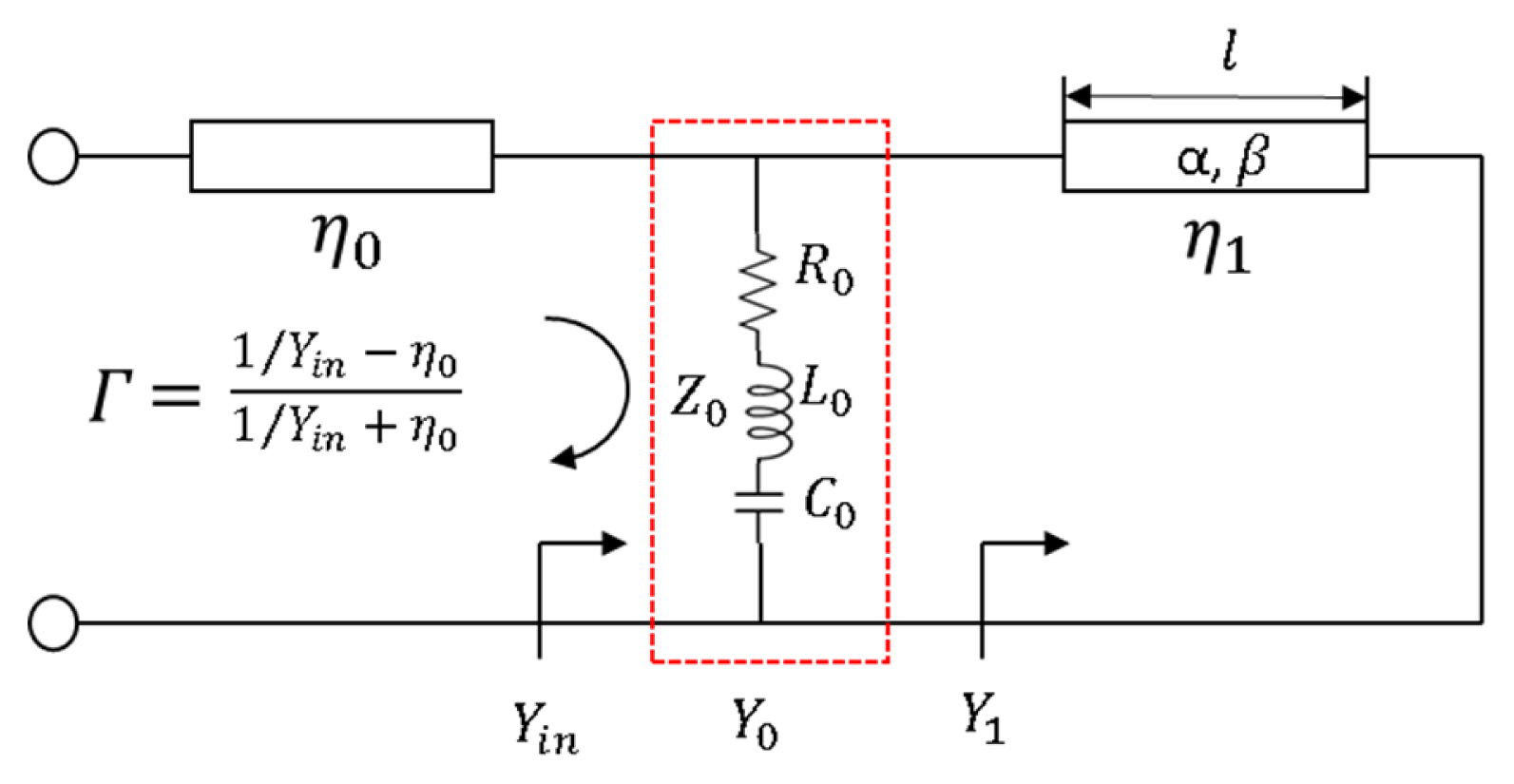
Fig. 1 shows the geometry of a single-layer microwave absorber using a reactive RLC screen, which is realized by a periodic cross-dipole structure placed above a conducting plane. The permittivity of the substrate is ɛr. A simple dipole-type structure on a AgNW resistive film is used as a reactive screen. Fig. 2 is the equivalent circuit of Fig. 1 when the EM wave is normally incident. The dipole-shaped structure is modelled as a lumped series RLC resonator characterized by of R0, L0, and C0. In the equivalent circuit, η0 (= 377 Ω) and η1 are the intrinsic impedances of free space and the substrate, respectively. Z0 is the impedance of the RLC reactive screen alone. Y1 is the input admittance at the input terminal of the shorted transmission line with length l. The characteristic impedance of the transmission line is η1. α is the attenuation constant, and β is the propagation constant. Yin is the total input admittance of the RLC screen. If the electrical length βl at a design angular frequency ω0 and intrinsic impedance η1, with the permittivity of the substrate are specified, the RLC values (R0, L0, and C0) can be determined and used to obtain the dimensions of the dipole structure through EM simulations, as shown in Section III.
In Fig. 2, the admittance Y0 of the RLC screen is
The input admittance Y1 of the shorted transmission line is
where l is the physical length, and γ = α + jβ is a complex propagation constant of a lossy line. The attenuation constant α can be written in terms of loss tangent (tanδ). αl is given by
and βl is given by
β l = θ 0 ω ω 0
The input admittance Yin as a function of frequency is given by
The reflection coefficient Γ and the absorption A are given by
and
The condition of perfect absorption (A = 1) at ω0 is that Eq. (5) is zero at ω0. This condition results in
and
One more chosen condition to uniquely determine R0, L0, and C0 is that the slope of the imaginary part (Bin) of input admittance (4) should be 0 at ω = ω0 [24], given by
If the differentiation of Bin is 0 (9) and satisfies (7) and (8) at ω0, it is guaranteed that the absorption is perfect at ω0 and has the widest bandwidth possible. Note that the real part (Gin) of input admittance (4) is flatter than Bin near ω0, especially when θ0 approaches 90°. Eq. (9) is expressed as follows:
where
and
Eqs. (7), (8), and (10) are simultaneously solved for R0, L0, and C0. The resistance R0 in Fig. 2 is given by
The inductance L0 and capacitance C0 can be obtained from the two equations as follows:
and
and
The closed-form solutions for R0, L0, and C0 are all related to the intrinsic impedance (η1), loss tangent (tanδ), and electrical thickness (θ0) (at ω0) of the substrate.
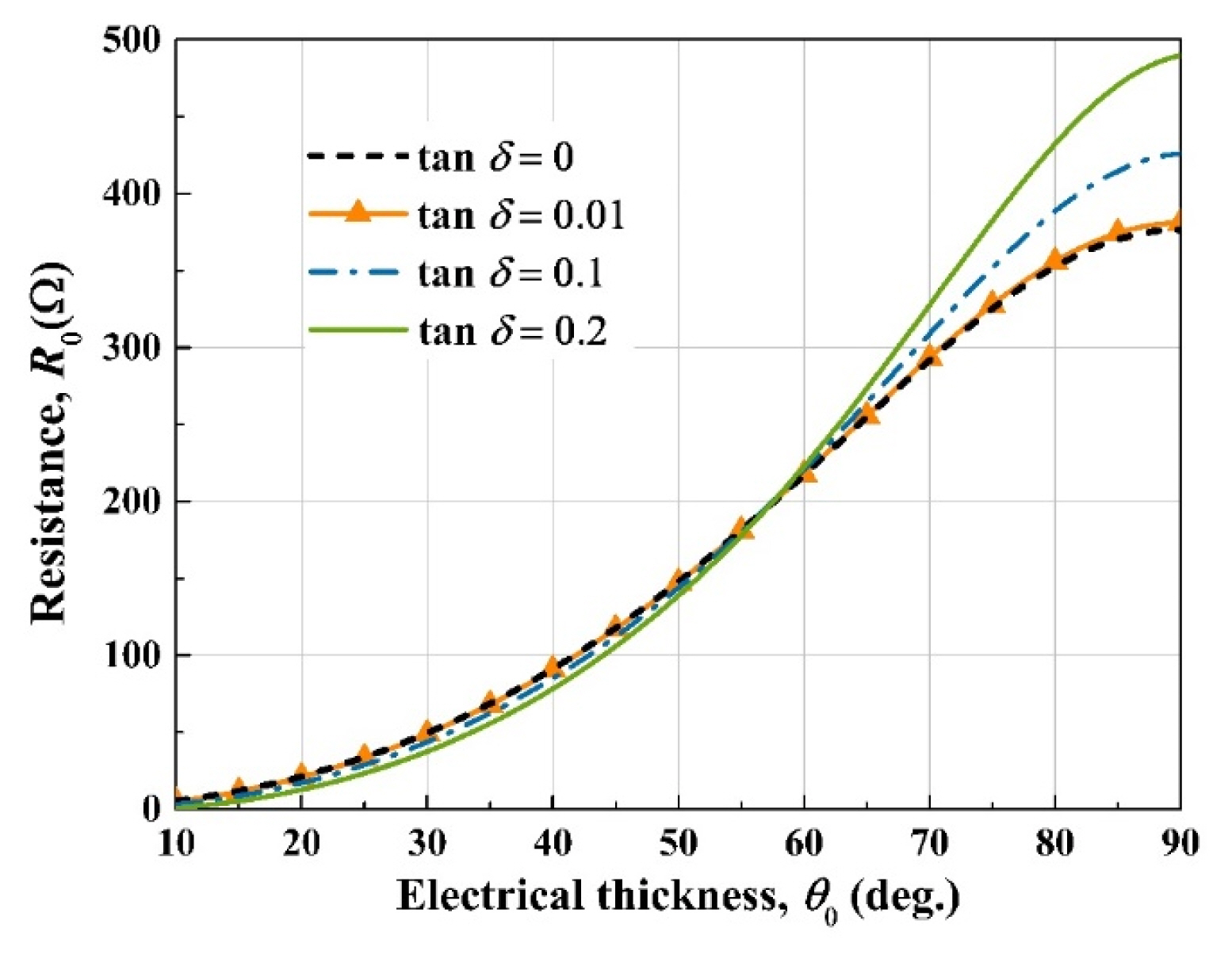
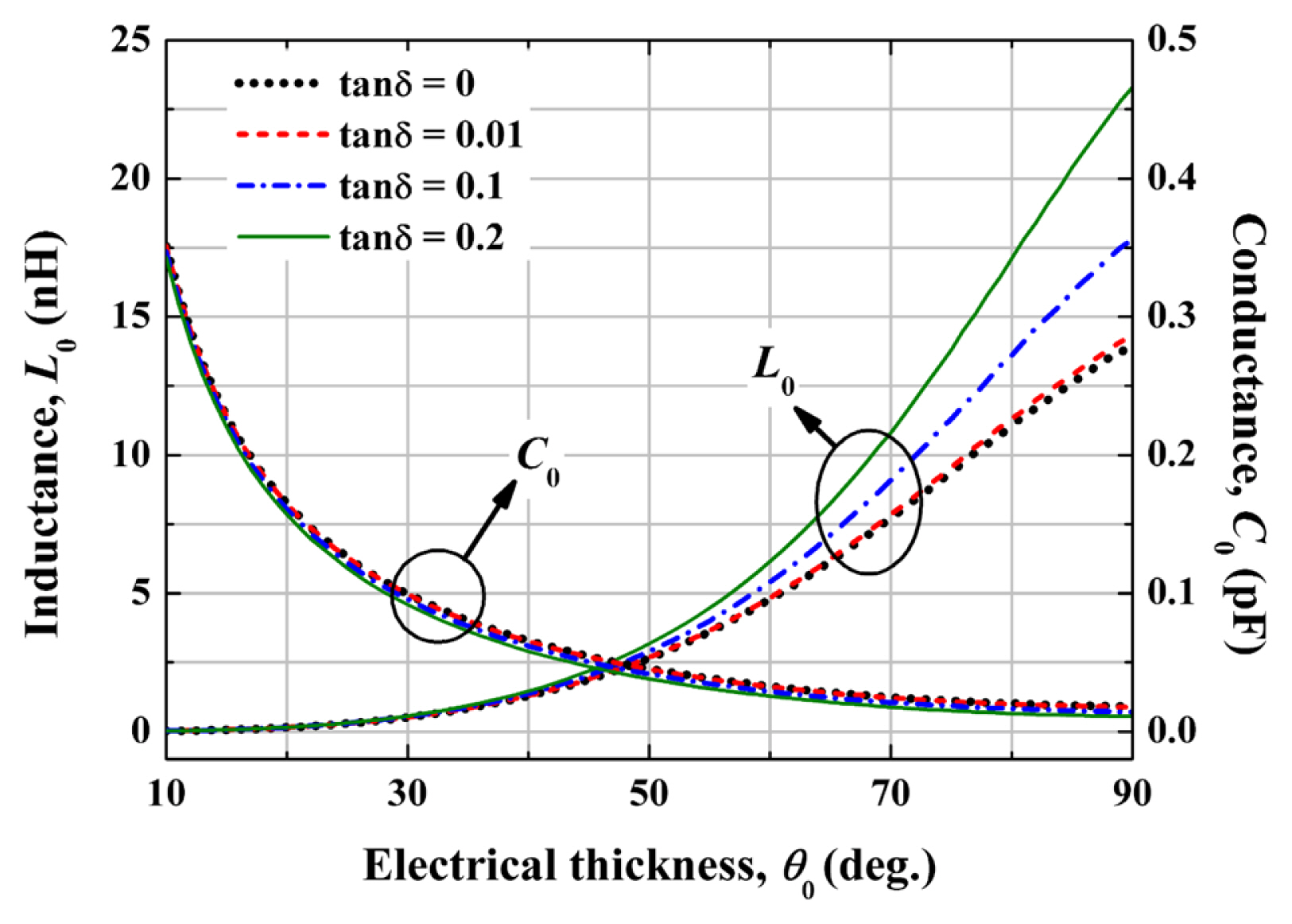
The values of R0 (13) with different electrical thicknesses and loss tangents are shown in Fig. 3. As the electrical thickness θ0 becomes larger, the value of R0 also becomes larger. When the loss tangent is smaller than 0.01, the value of R0 is almost the same as in the case of no loss. The effects of loss on the substrate are shown to be more pronounced when θ0 is greater than 70°. Note that, when the loss tangent is zero and θ0 is 90°, R0 approaches 377 Ω which is same as Salisbury’s. The values of L0 (16) and C0 (17) with different electrical thicknesses and loss tangents are plotted in Fig. 4. The effect of the loss tangent is not significant in C0 but is relatively more significant in L0, especially when θ0 is greater than 70°.
When the loss tangent of the substrate is assumed to be zero, solutions (13) and (16)–(17) are simplified to
and
Especially when θ0 is 90°, the above solutions are simplified again to those in [5]. Thus, we can see that the solutions presented in this work are general and can be applied to any thickness, permittivity, loss tangent of the substrate, and frequency.
Fig. 5 shows the absorption (6) for different electrical thicknesses of the absorber. The widest bandwidth is obtained when θ0 is 70°. The 90% (A = 0.9) bandwidth is approximately 8 GHz, from 8.2 GHz to 16.2 GHz (66% at a center frequency of 12.2 GHz or 80% at a design frequency of 10 GHz).
Based on these observations, the specifications of the desired absorber are summarized in Table 1. The design frequency is 10 GHz for X-band absorber applications. The relative permittivity and loss tangent of the substrate are 2.2 and 0.001, respectively. The obtained values of R0, L0, and C0 using (13) and (16)–(17) are shown in Table 2.
The absorber with crossed-dipole patterns (Fig. 1) based on the parameters shown in Tables 1 and 2 is realized using an EM simulator (High Frequency Simulation Software), fabricated, and measured. The reason why the crossed-dipole pattern is chosen among other structures is that it is the simplest and easiest to implement. Moreover, its absorption characteristics are almost independent of the polarization of incident waves, as will be shown later.
Fig. 6 shows the geometry of the absorber unit cell. a is the side length of the square absorber unit, and w and h are the width and height, respectively, of the crossed-dipole pattern on a resistive film. Rs is the resistance per square of the film material, and g is the gap distance. To realize the real part (R0 = 291.86 Ω) of the reactive screen, a AgNW film with 23 Ω/square is used. AgNWs are chosen for fabrication because the sheet resistance of the film can be easily controlled by adjusting the concentration of the AgNW solution. The width (w) and length (h) of the dipole structure affect the resistance R0, which is given by Rsh/w, where Rs is the surface resistance of the resistive sheet (Ω/square). By choosing Rs and the aspect ratio h/w properly, a specifically required R0 can be realized in many ways. Moreover, L0 and C0 are proportional to h/w and w/g, respectively. By using these characteristics, the overall dimensions of the crossed-dipole structure can be determined using an EM simulator.
The first step is to determine the dimensions of the crossed-dipole in which the circuit values in Table 2 are realized. To evaluate the impedance of only the reactive screen in HFSS, deembedding from the ports to the reactive screen is performed, as shown in Fig. 7(a). After the realization of the structure on the reactive screen, the substrate and ground are added below the reactive screen, as shown in Fig. 7(b). The final dimensions of the absorber are listed in Table 3.
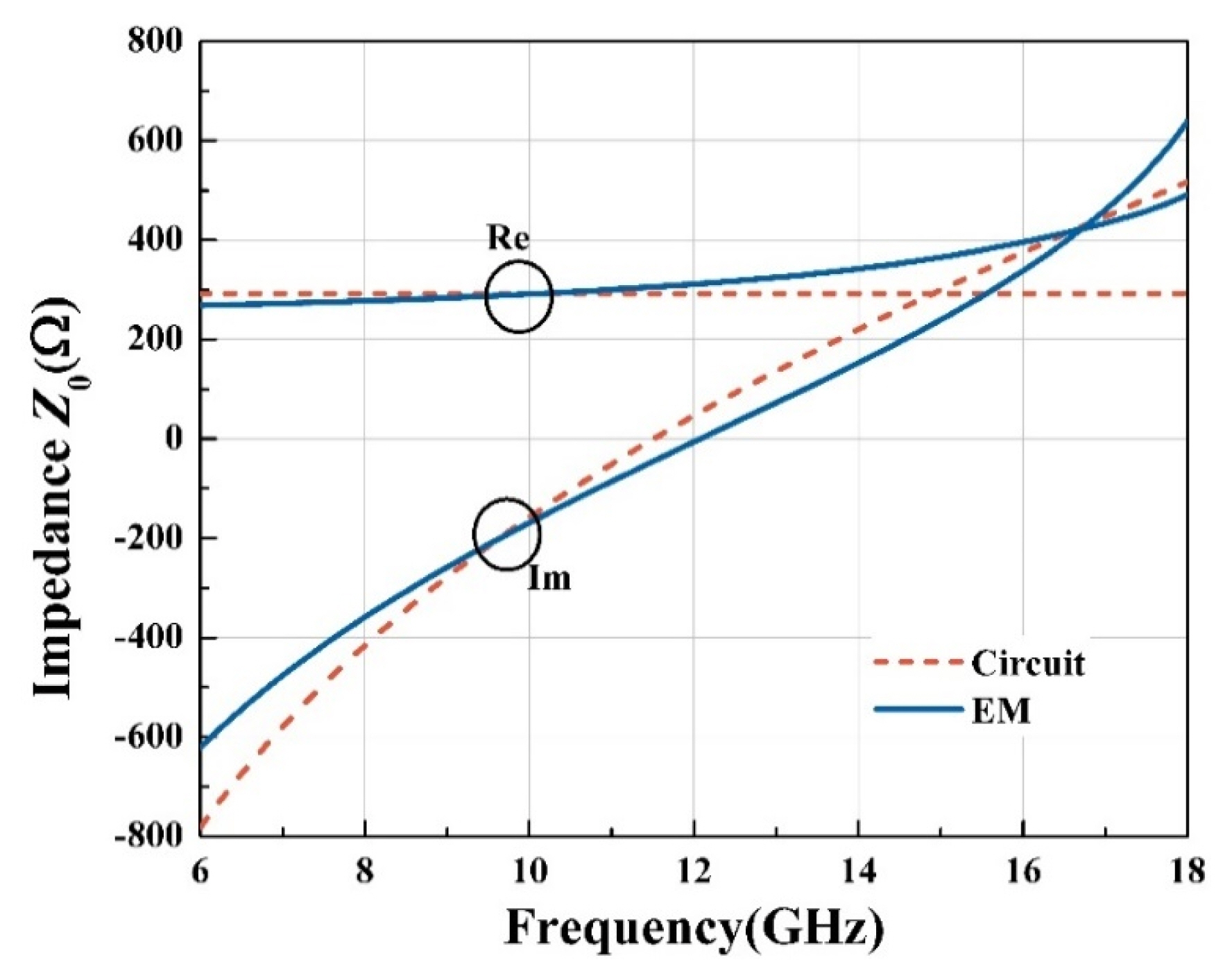
Fig. 8 shows the resistance and reactance of the impedance only for the reactive RLC screen based on circuit and EM simulations. The circuit simulation results are used as a reference in determining the dimensions of the crossed-dipole structure. Even though the lumped circuit values in Table 2 are used in the circuit simulation, and the crossed-dipole in the unit cell size of 0.37λ0 is not smaller than the wavelength, a good resemblance between them is observed. The input impedance Zin, as defined in Fig. 2 and simulated based on Fig. 7(b), is shown in Fig. 9 as a function of the frequency based on the EM and circuit simulations. Without any adjustment to the designed crossed-dipole structure, the EM simulation results reasonably agree with the circuit simulation results. The circuit modelling of the crossed-dipole structure (0.37λ0 in Table 3) by the lumped elements R0, L0, and C0 is expected to intrinsically lead to some discrepancies between the circuit and EM simulations. Despite these discrepancies, the design guidance by circuit modelling is helpful. Note that the imaginary part is zero (resonant) with a flat slope, and the real part is 377 Ω. This enables perfect absorption (or match) at the design frequency and maximum bandwidth.
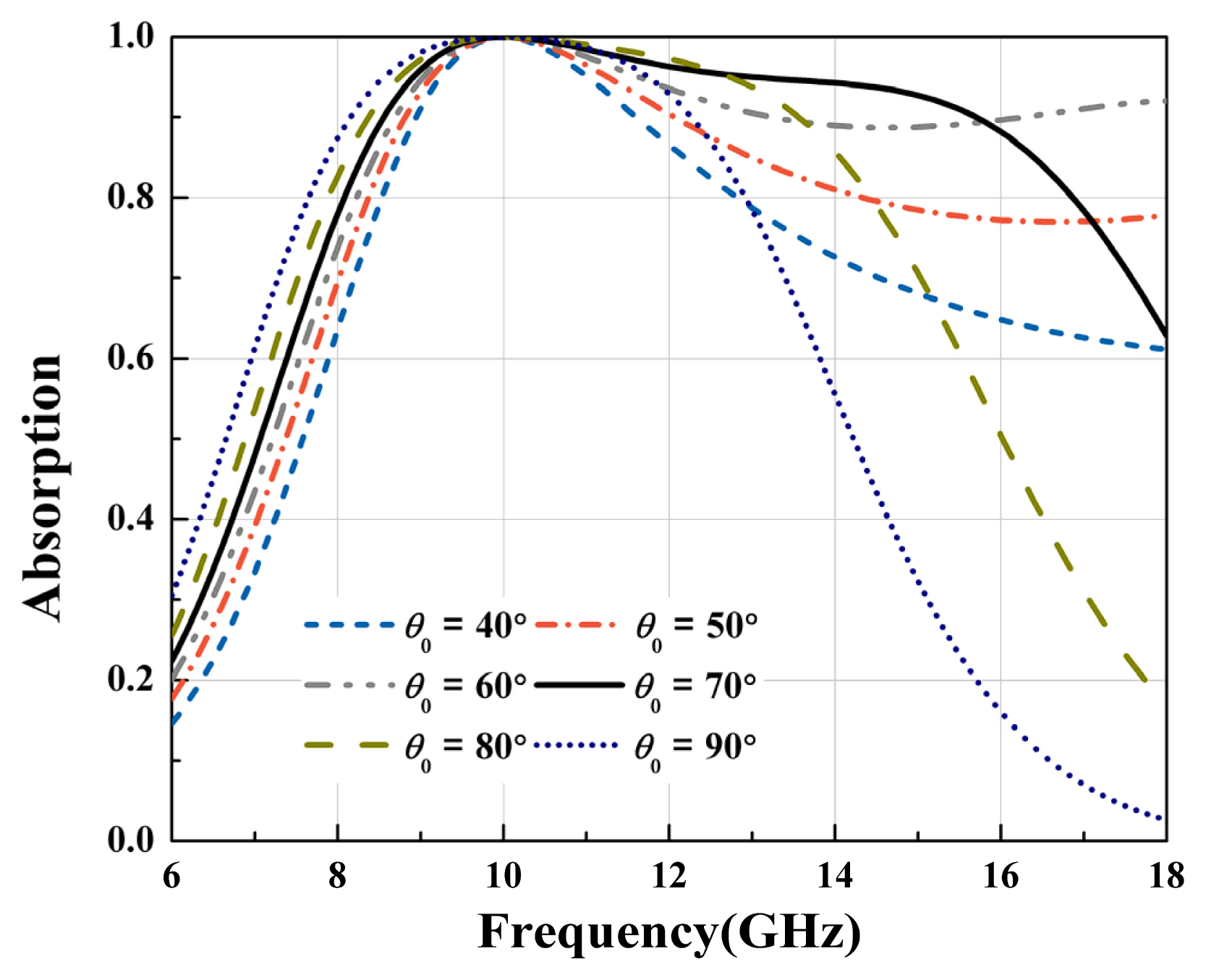
Fig. 10 shows the EM-simulated absorptions depending on the polarization angles (φ) of 0°–45°. The absorption is almost independent of the polarization angle because of the crossed-dipole structure employed. The degradation effects of microwave absorbers with oblique TE and TM incidence cases are mostly similar to those in [7].
Fig. 11(a) is a photograph of the fabricated absorber made of a AgNW resistive film, a substrate, and a conducting plane. The absorber consists of 25 × 17 unit cells, and the overall size is 270.5 mm × 183.9 mm × 4.5 mm. The experimental setup is illustrated in Fig. 11(b). Absorption was measured using a high-gain horn antenna of Model BBHA9120D (1–18 GHz; Schwarzbeck, Schönau, Germany). Fig. 12 shows the measured absorption compared with the circuit and EM simulation results. The measured absorption in Fig. 12 was obtained based on a special calibration for the network analyzer and with the use of a conducting plane at the back of the absorber as a reference [6]. The distance between the horn antenna and the absorber is 5λ0 (15 cm) at 10 GHz. The 3 dB beamwidth (in the E and H planes) of the horn antenna is approximately 45° at 10 GHz. Some variations of this distance (5λ0) do not affect the measured reflection coefficient and absorption at the absorber. The absorption results are shown to reasonably agree with each other. Some differences between the simulations and the measurement could have resulted from the non-uniformity of the resistivity in the AgNW film and the limited size of the absorber in the measurement. According to the specification, the surface resistance of the AgNW resistive film (Kolon Industries, Seoul, Korea) is 23 Ω/square ±4%.
Closed-form solutions for the design of thin and wideband microwave absorbers have been derived and presented based on an equivalent circuit. These solutions can be used for any design frequency, substrate thickness, and permittivity/loss tangent. As a design example, a microwave absorber with a design center frequency of 10 GHz with 70° electrical thickness has been designed using the derived solutions and fabricated using a AgNW resistive film with a surface resistance of 23 Ω/square. The measured absorption shows consistent results with the circuit (theory) and EM simulations, validating the effectiveness of the proposed design procedures. A thin and wideband absorber can now be more easily designed without unnecessary trial-and-error efforts or complicated processes.
Acknowledgments
This research was supported by the MSIT (Ministry of Science and ICT), Korea, under the Information Technology Research Center support program (No. IITP-2021-2016-0-00291) supervised by the Institute for Information & Communications Technology Planning & Evaluation (IITP).
Fig. 6
Geometry of the absorber unit cell using a resistive crossed-dipole pattern for a reactive RLC screen.
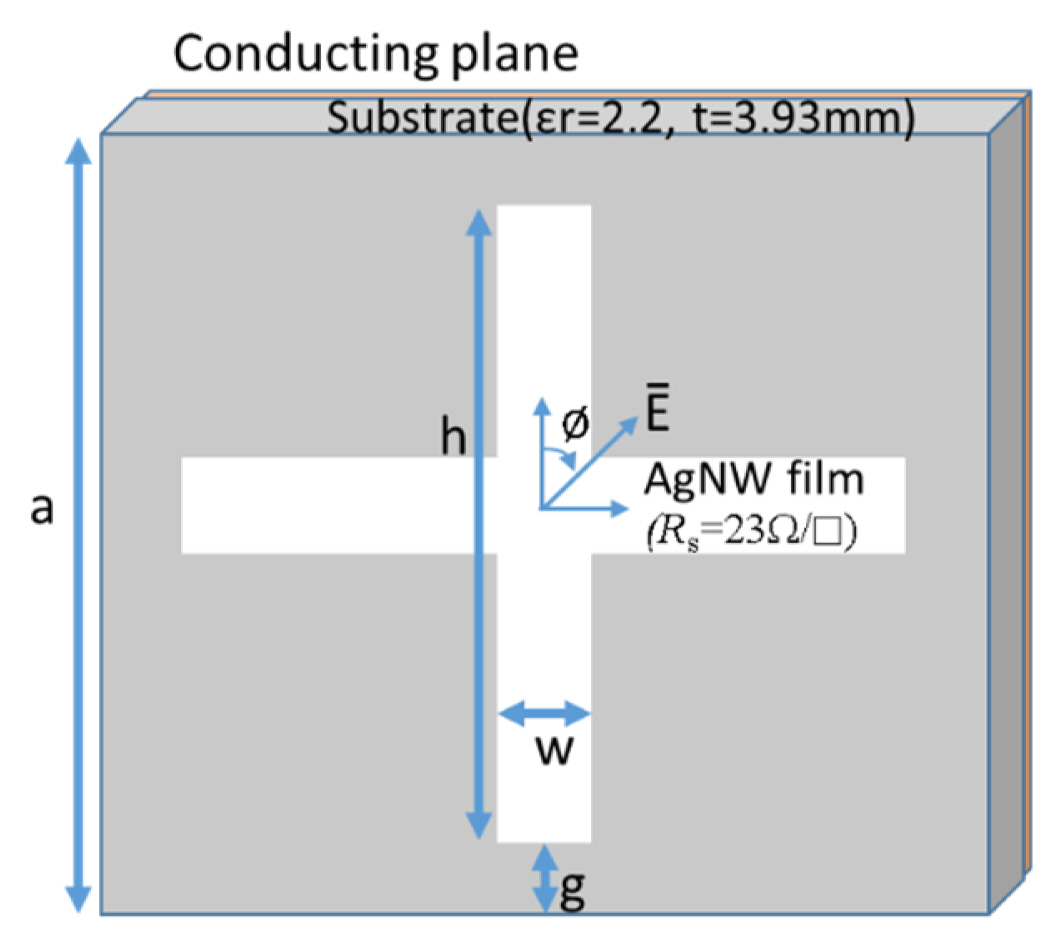
Fig. 7
Configuration of the EM simulation to evaluate (a) the impedance of the reactive screen using de-embedding and (b) the reflection coefficient and absorption from the absorber.
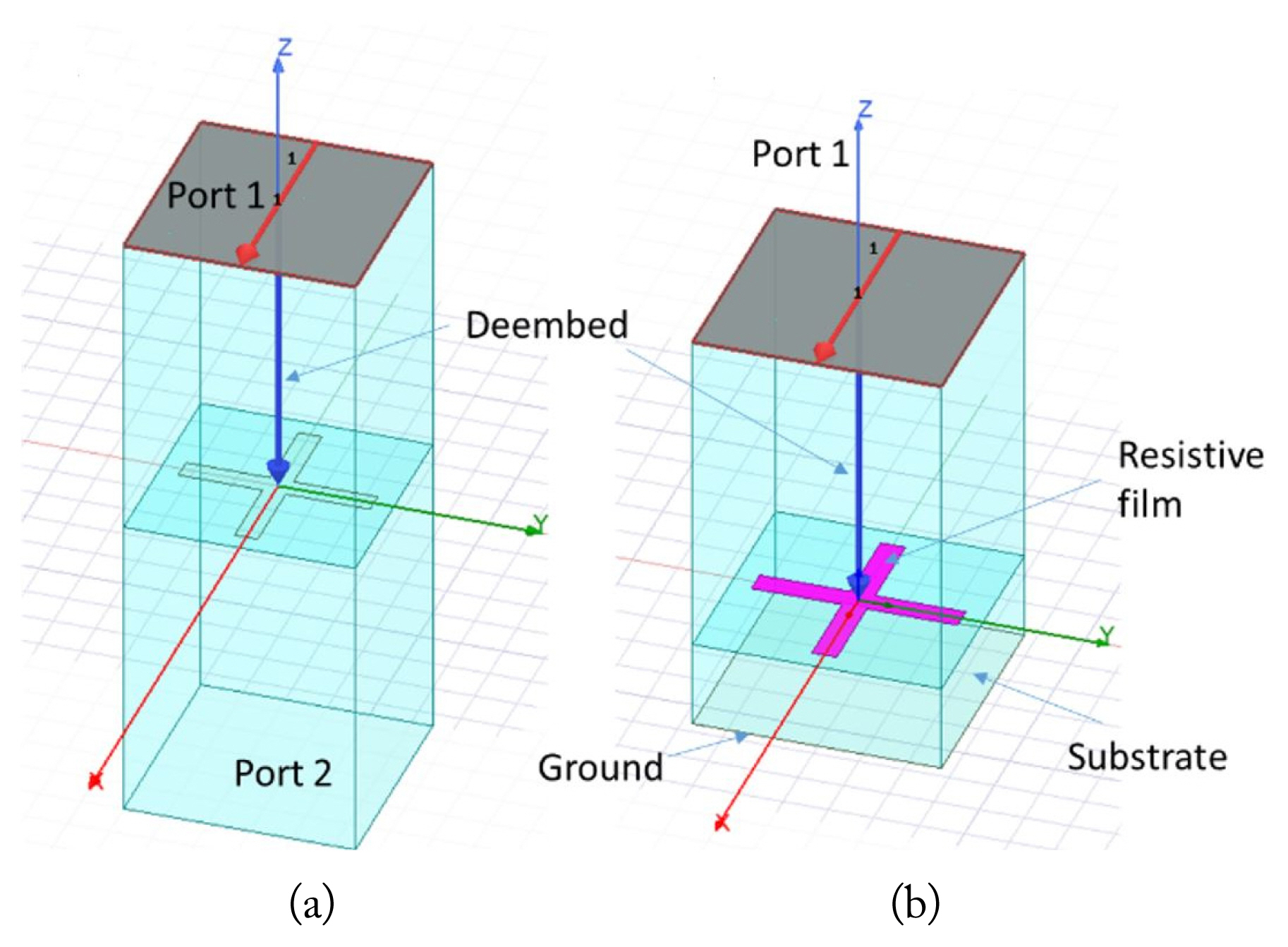
Fig. 9
Input impedance Zin (Fig. 2) as a function of frequency based on the EM and circuit simulations.
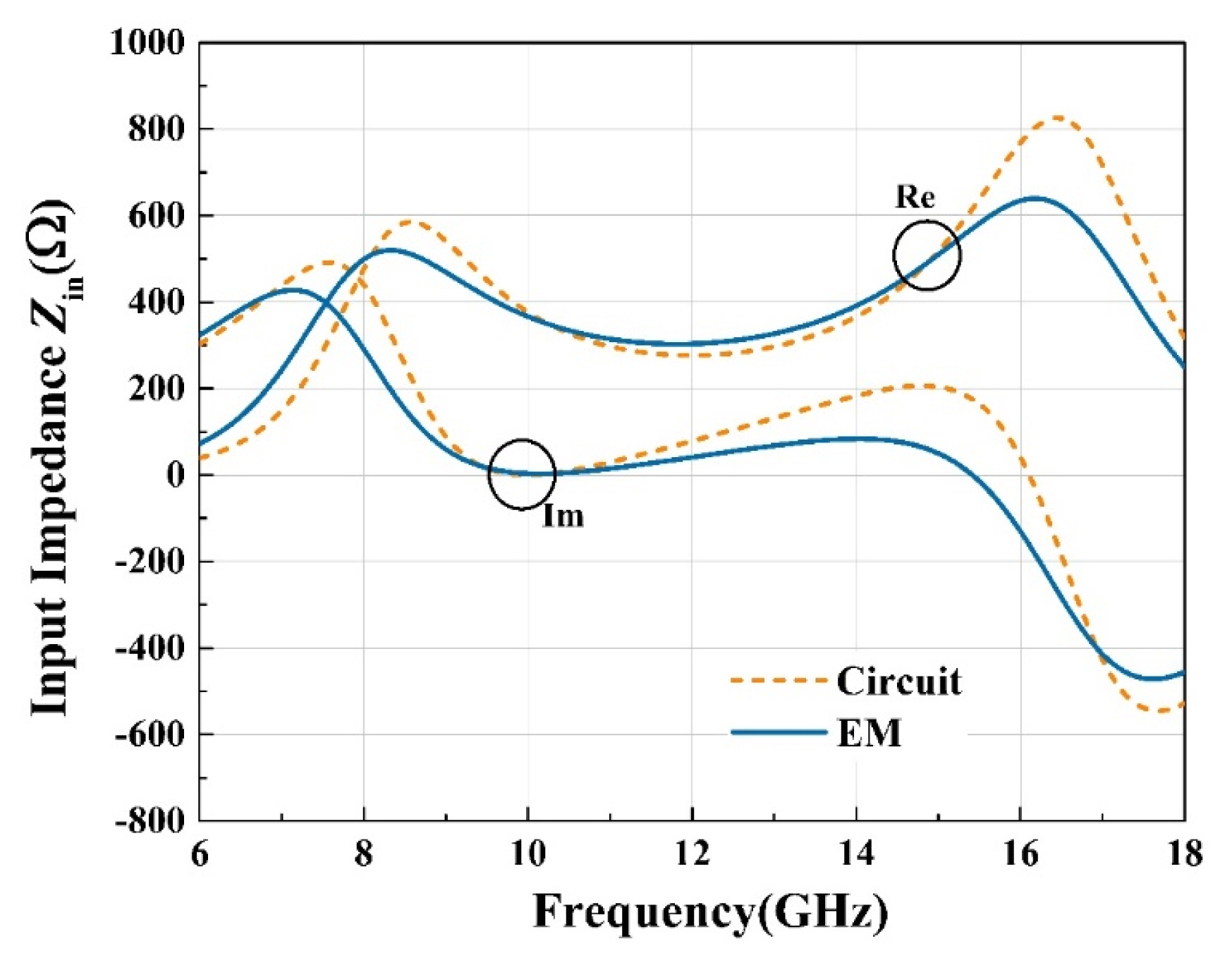
Fig. 10
EM-simulated absorptions of the proposed absorber as a function of frequency depending on the polarization angle (φ) defined in Fig. 6.

Fig. 11
Photograph of (a) the crossed-dipole reactive screen realized on a AgNW film and (b) the experimental setup. Insets (a) and (b) show the enlarged views of the crossed-dipole structure and the absorber/horn antenna.

Fig. 12
Measured absorption of the fabricated absorber compared with the circuit and EM simulation results.
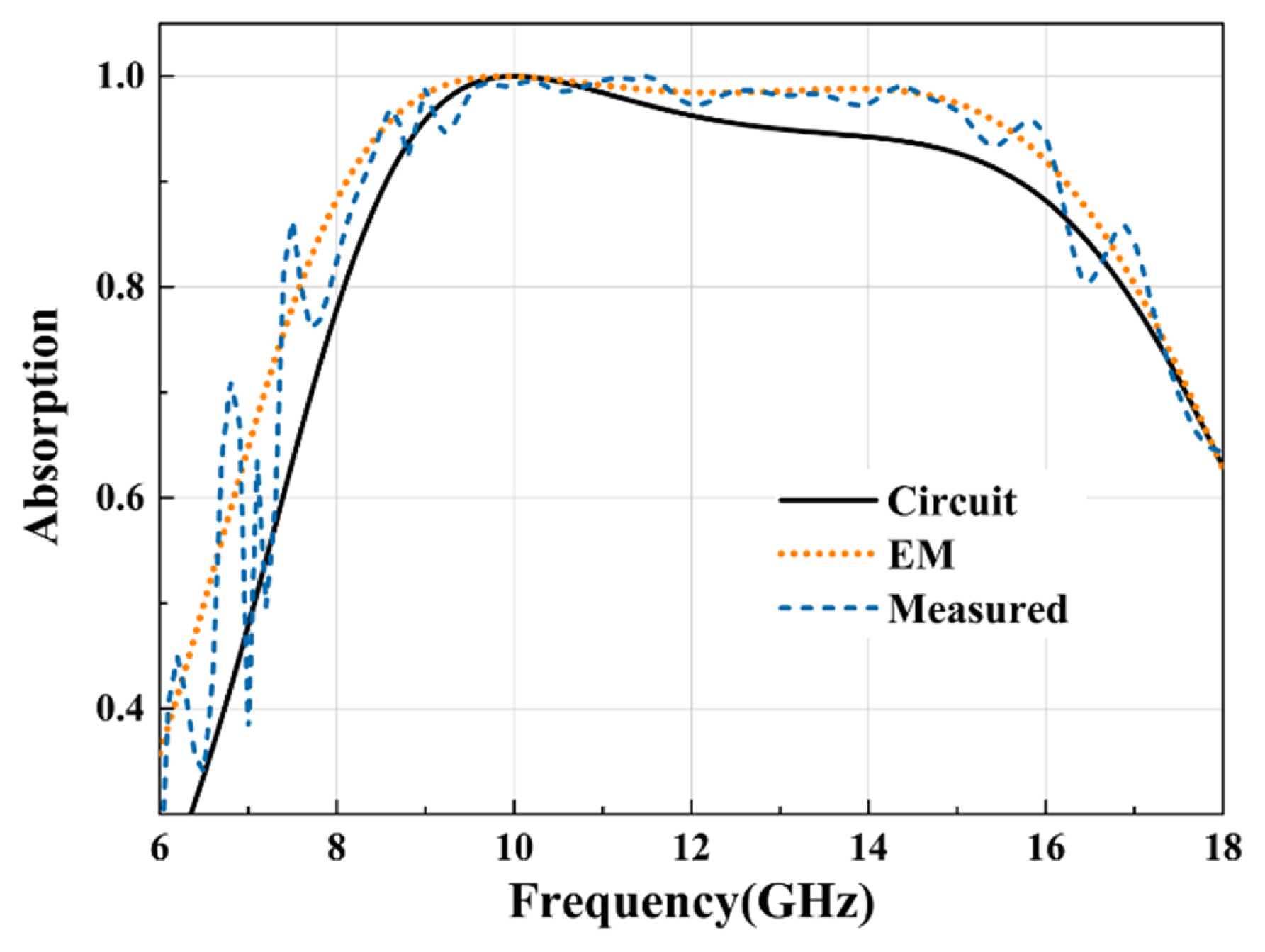
Table 1
Parameters of the absorber design
| Parameter | Value |
|---|---|
| Frequency (f0) | 10 GHz |
| Permittivity (ɛr) | 2.2 |
| Thickness (electrical length) | 3.93 mm (θ0 = 70°) |
| Tangential loss for substrate | 0.001 |
Table 2
The values of R0, L0, and C0 for the absorber described in Table 1
| Circuit value | Value |
|---|---|
| R0 | 291.86 Ω |
| L0 | 7.73 nH |
| C0 | 24.74 fF |
References
1. WW Salisbury, "Absorbent body for electromagnetic waves," US Patent, 2599944. Jun 10 1952.
2. RL Fante and MT McCormack, "Reflection properties of the Salisbury screen," IEEE Transactions on Antennas and Propagation, vol. 36, no. 10, pp. 1443–1454, 1988.

3. BA Munk, Frequency Selective Surfaces, Theory and Design. New York, NY: Wiley, 2000.
4. G Kim and B Lee, "Design of wideband absorbers using RLC screen," Electronics Letters, vol. 51, no. 11, pp. 834–836, 2015.

5. G Kim, S Kim, and B Lee, "Design of wideband microwave absorbers using reactive Salisbury screens with maximum flat reflection," Journal of Electromagnetic Engineering and Science, vol. 19, no. 2, pp. 71–81, 2019.

6. BK Kim and B Lee, "Wideband absorber at X-band adopting trumpet-shaped structures," Electronics Letters, vol. 50, no. 25, pp. 1957–1959, 2014.

7. BA Munk, P Munk, and J Pryor, "On designing Jaumann and circuit analog absorbers (CA absorbers) for oblique angle of incidence," IEEE Transactions on Antennas and Propagation, vol. 55, no. 1, pp. 186–193, 2007.

8. T Deng, Y Yu, Z Shen, and ZN Chen, "Design of 3-D multilayer ferrite-loaded frequency-selective rasorbers with wide absorption bands," IEEE Transactions on Microwave Theory and Techniques, vol. 67, no. 1, pp. 108–117, 2019.

9. M Li, S Xiao, YY Bai, and BZ Wang, "An ultrathin and broadband radar absorber using resistive FSS," IEEE Antennas and Wireless Propagation Letters, vol. 11, pp. 748–751, 2012.

10. GR Zhang, PH Zhou, HB Zhang, LB Zhang, JL Xie, and LJ Deng, "Analysis and design of triple-band high-impedance surface absorber with periodic diversified impedance," Journal of Applied Physics, vol. 114, article no. 164103, 2013.
https://doi.org/10.1063/1.4826265

11. Y Shang, Z Shen, and S Xiao, "On the design of single-layer circuit analog absorber using double-square-loop array," IEEE Transactions on Antennas and Propagation, vol. 61, no. 12, pp. 6022–6029, 2013.

12. V Mishra, R Panwar, A Singh, S Puthucheri, and D Singh, "Critical analysis of periodic fractal frequency selective surfaces coupled with synthesised ferrite-based dielectric substrates for optimal radar wave absorption," IET Science, Measurement & Technology, vol. 13, no. 6, pp. 794–802, 2019.

13. R Panwar, S Puthucheri, D Singh, and V Agarwala, "Design of ferrite–graphene-based thin broadband radar wave absorber for stealth application," IEEE Transactions on Magnetics, vol. 51, no. 11, pp. 1–4, 2015.



14. S Isha, M Najim, P Smitha, D Singh, and GD Varma, "Microwave absorption properties of nanostructured nickel ferrite," In: Proceedings of 2014 International Conference on Electronics and Communication Systems (ICECS); Coimbatore, India, 2014, pp 1–4.

15. AP Sohrab and Z Atlasbaf, "A circuit analog absorber with optimum thickness and response in X-band," IEEE Antennas and Wireless Propagation Letters, vol. 12, pp. 276–279, 2013.

16. D Kundu, A Mohan, and A Chakrabarty, "Single-layer wideband microwave absorber using array of crossed dipoles," IEEE Antennas and Wireless Propagation Letters, vol. 15, pp. 1589–1592, 2016.

17. Y Han, W Che, C Christopoulos, Y Xiong, and Y Chang, "A fast and efficient design method for circuit analog absorbers consisting of resistive square-loop arrays," IEEE Transactions on Electromagnetic Compatibility, vol. 58, no. 3, pp. 747–757, 2016.

18. D Singh, A Kumar, S Meena, and Y Agarwala, "Analysis of frequency selective surfaces for radar absorbing materials," Progress In Electromagnetics Research, vol. 38, pp. 297–314, 2012.

19. Z Zhou, K Chen, J Zhao, P Chen, T Jiang, B Zhu, Y Feng, and Y Li, "Metasurface Salisbury screen: achieving ultra-wideband microwave absorption," Optics Express, vol. 25, no. 24, pp. 30241–30252, 2017.


20. A Sharma, R Panwar, and R Khanna, "Experimental validation of a frequency-selective surface-loaded hybrid metamaterial absorber with wide bandwidth," IEEE Magnetics Letters, vol. 10, article no. 2101905, 2019.
https://doi.org/10.1109/LMAG.2019.2898612

21. JBO de Araujo, GL Siqueira, E Kemptner, M Weber, C Junqueira, and MM Mosso, "An ultrathin and ultrawideband metamaterial absorber and an equivalent-circuit parameter retrieval method," IEEE Transactions on Antennas and Propagation, vol. 68, no. 5, pp. 3739–3746, 2020.

22. YQ Pang, YJ Zhou, and J Wang, "Equivalent circuit method analysis of the influence of frequency selective surface resistance on the frequency response of metamaterial absorbers," Journal of Applied Physics, vol. 110, article no. 023704, 2011.
https://doi.org/10.1063/1.3608169

23. DM Pozar, Microwave Engineering. Hoboken, NJ: John Wiley & Sons Inc, 2012. p.96–102.
24. TH Lee, Planar Microwave Engineering: A Practical Guide to Theory, Measurement, and Circuits. Cambridge, UK: Cambridge University Press, 2004.
Biography

Myungwan Lyou received a B.S. degree in Information & Communication Engineering from Hoseo University, Korea, in 1998 and M.S. degree in Radio Engineering from Kyung Hee University, in 2000. He is currently working toward a Ph.D. degree in Electronics Engineering at Kyung Hee University from 2013. He has been working for KTC (Korea Testing Certification Institute) in Korea since 2001. His fields of research include microwave antennas, passive devices, EMC, and absorbers.
Biography

Gunyoung Kim received a B.S. degree in Radio Communication Engineering from Kyung Hee University, Yongin, Korea, in 2010. M.S. and Ph.D. degree in Electronics and Radio Engineering from Kyung Hee University, 2012 and 2019. He is currently working for Hanwha Systems in Korea from 2020. His fields of research include microwave antennas, passive devices, wireless power transmission, and metamaterials.
Biography

Bomson Lee received the B.S. degree in Electrical Engineering from Seoul National University, Seoul, Korea, in 1982. From 1982 to 1988, he was with the Hyundai Engineering Company Ltd., Seoul, Korea. He received the M.S. and Ph.D. degrees in Electrical Engineering from the University of Nebraska, Lincoln, NE, U.S.A., in 1991 and 1995, respectively. In 1995, he joined the faculty at Kyung Hee University, where he is currently a professor in the Department of Electronics and Radio Engineering. From 2007 to 2008, he was the chair of the technical group for microwave and radio wave propagation in KIEES (Korea Institute of Electromagnetic Engineering & Science). In 2010, he was an editor- in-chief of the Journal of the Korean Institute of Electromagnetic Engineering and Science. From 2015 to 2016, he has been a vice-chairman in KIEES. In 2017, he has been an executive vice-chairman in KIEES. In 2018, he served as the president in KIEES. His research activities include microwave antennas, RF identification (RFID) tags, microwave passive devices, wireless power transfer, and metamaterials.
- TOOLS